Heap Sort
- 최대 힙 트리나 최소 힙 트리를 구성해 정렬을 하는 방법입니다.
- 내림차순 정렬을 위해서는 최대 힙을 구성하고 오름차순 정렬을 위해서는 최소 힙을 구성하면 됩니다.
- 과정
- 정렬해야 할 n개의 요소들로 최대 힙(완전 이진 트리 형태)를 만듭니다.
- 그 다음에 한 번에 하나씩 요소를 힙에서 꺼내서 배열의 뒤부터 저장하면 됩니다.
- 삭제되는 요소들(최댓값부터 삭제)는 값이 감소되는 순서로 정렬되게 됩니다.
max Heap을 삭제하고 추가하는 과정은 이미 안다고 가정하고 코드를 바로 짜 보도록 하겠습니다.
아래 코드는 Max Heap을 구현한 다음에 최댓값 부터 pop해 가면서 배열의 뒷 쪽 부터 삽입해 가는 코드라고 보시면 됩니다.
/heap_sort.c
#include <stdio.h> #include <stdlib.h> #include <string.h> #define ARR_SIZE 100 #define HEAP_FULL(n) (n == ARR_SIZE - 1) #define HEAP_EMPTY(n) (!n) int sorted[ARR_SIZE]; typedef struct { int key; } element; element heap[ARR_SIZE]; void push(element item, int *n) { int i; // 만약 힙이 꽉 차있다면 if (HEAP_FULL(*n)) { fprintf(stderr, "The heap is full. \n"); exit(EXIT_FAILURE); } i = ++(*n); while ((i != 1) && (item.key > heap[i / 2].key)) { heap[i] = heap[i / 2]; i /= 2; } heap[i] = item; } element pop(int *n) { int parent, child; element item, temp; if (HEAP_EMPTY(*n)) { fprintf(stderr, "The heap is empty. \n"); exit(EXIT_FAILURE); } item = heap[1]; temp = heap[(*n)--]; parent = 1; child = 2; while (child <= *n) { if ((child < *n) && (heap[child].key < heap[child + 1].key)) child++; if (temp.key >= heap[child].key) break; heap[parent] = heap[child]; parent = child; child *= 2; } heap[parent] = temp; return item; } void heap_sort(int a[], int n) { int count = 0, i; element newElement = {0}; // 우선 int 배열의 값을 하나씩 heap에 집어 넣어주어야 한다. for (int i = 0; i < n; i++) { newElement.key = a[i]; push(newElement, &count); } for (i = (n - 1); i >= 0; i--) { a[i] = pop(&count).key; } } int seqSearch(int a[], int k, int n) { int i; for (i = 1; i < n && a[i] != k; i++) ; if (i >= n) return -1; return i; } void swap(int *a, int *b) { int temp; temp = *a; *a = *b; *b = temp; } void insertionSort(int a[], int n) { /* sort a[0:n - 1] into nondecreasing order( 오름 차순 ) */ int i, j, key; for (i = 1; i < n; i++) { key = a[i]; // 현재 정렬된 배열은 i - 1까지 이므로 i - 1번째부터 역순으로 조사합니다. for (j = i - 1; j >= 0 && a[j] > key; j--) a[j + 1] = a[j]; a[j + 1] = key; } } int partition(int list[], int left, int right) { int pivot; int low, high; low = left; high = right + 1; pivot = list[left]; // 정렬할 리스트의 가장 왼쪽 데이터를 피벗으로 선택 ( 임의의 값을 피벗으로 선택 ) /* low와 high가 교차할 때까지 반복 (low < high) */ do { /* list[low]가 피벗보다 작으면 계속 low를 증가 시켜줘야 한다. */ do { low++; } while (list[low] < pivot && low <= right); // low가 만약에 right이 된다면 멈처 주어야 합니다. do { high--; } while (list[high] > pivot && high >= left); // 만약 low와 high가 교차하지 않았으면 list[low]를 list[high]와 교환 if (low < high) swap(list + low, list + high); } while (low < high); // low와 high가 교차했으면 반복문을 빠져나와 list[left]와 list[high]을 교환해줘야 한다. swap(list + left, list + high); // 피벗의 위치인 high를 반환해주어야 합니다. return high; } void quick_sort(int list[], int left, int right) { /* 정렬할 범위가 2개 이상의 데이터가렴( 리스트의 크기가 0이나 1이 아니라면) */ if (left < right) { // partition함수를 호출하여 피벗을 기준으로 리스트를 비균등 분할 - 분할(Divide) int q = partition(list, left, right); // 피벗을 제외한 2개의 부분 리스트를 대상으로 순환 호출 quick_sort(list, left, q - 1); // (left ~ 피벗 바로 앞) 앞쪽 부분 리스트 정렬 - 정복(Conquer) quick_sort(list, q + 1, right); // (피벗 바로 뒤 ~ right) 뒤쪽 부분 리스트 정렬 - 정복(Conquer) } else { return; } } void merge(int list[], int left, int mid, int right) { int i, j, k, l; i = left; j = mid + 1; k = left; /* 분할 정렬된 list의 합병 */ while (i <= mid && j <= right) { if (list[i] <= list[j]) sorted[k++] = list[i++]; else sorted[k++] = list[j++]; } // 남아 있는 값들을 일괄 복사 if (i > mid) for (l = j; j <= right; j++) sorted[k++] = list[j]; else for (l = i; i <= mid; i++) sorted[k++] = list[l]; for (l = left; l <= right; l++) list[l] = sorted[l]; } void merge_sort(int list[], int left, int right) { int mid; // 원소가 1개가 아닌 경우 if (left < right) { mid = (left + right) / 2; // 중간 위치를 계산하여 리스트를 균등 분할 - 분할(Divide) merge_sort(list, left, mid); // 앞쪽 부분 리스트 정렬 - 정복(Conquer) merge_sort(list, mid + 1, right); // 뒤쪽 부분 리스트 정렬 - 정복(Conquer) merge(list, left, mid, right); // 정렬된 2개의 부분 배열을 합병하는 과정 - 결합(Combine) } else { // 원소가 1개라면 그냥 return시켜 주면 된다. return; } } void printArr(int a[], int n) { for (int i = 0; i < n; i++) printf("%d ", a[i]); putchar('\n'); } int main(void) { int sortArr[ARR_SIZE] = {0}; int count = 0; FILE *fp_read = fopen("sort.txt", "r"); while ((fscanf(fp_read, "%d", sortArr + count) != EOF)) { count++; } printf("original array: "); printArr(sortArr, count); // printf("[ 4 ] is in index %d\n", seqSearch(sortArr, 4, count)); int sortArr_2[ARR_SIZE]; memcpy(sortArr_2, sortArr, sizeof(int) * ARR_SIZE); printf("insertion sort:\t"); insertionSort(sortArr_2, count); printArr(sortArr_2, count); int sortArr_3[ARR_SIZE]; memcpy(sortArr_3, sortArr, sizeof(int) * ARR_SIZE); printf("quiuck sort:\t"); quick_sort(sortArr_3, 0, count - 1); printArr(sortArr_3, count); int sortArr_4[ARR_SIZE]; memcpy(sortArr_4, sortArr, sizeof(int) * ARR_SIZE); printf("merge sort:\t"); merge_sort(sortArr_4, 0, count - 1); printArr(sortArr_4, count); int sortArr_5[ARR_SIZE]; memcpy(sortArr_5, sortArr, sizeof(int) * ARR_SIZE); printf("heap sort:\t"); heap_sort(sortArr_5, count); printArr(sortArr_5, count); return 0; } // original array: 3 2 4 1 9 8 // insertion sort: 1 2 3 4 8 9 // quiuck sort: 1 2 3 4 8 9 // merge sort: 1 2 3 4 8 9 // heap sort: 1 2 3 4 8 9
- 장점
- 시간 복잡도가 좋은 편입니다.
- 힙 정렬이 가장 유용한 경우는 자료 전체를 정렬하는 것이 아니라 가장 큰 값 몇개만 필요할 때 입니다.
- 힙 트리의 전체 높이가 거의 logN(완전 이진 트리이므로)이므로 하나의 요소를 힙에 삽입하거나 삭제할 때 힙을 재정비하는 시간이 logN만큼 소요 됩니다.
- 요소의 개수가 N개이므로 전체적으로 O(NlogN)의 시간이 걸립니다.
총 정리
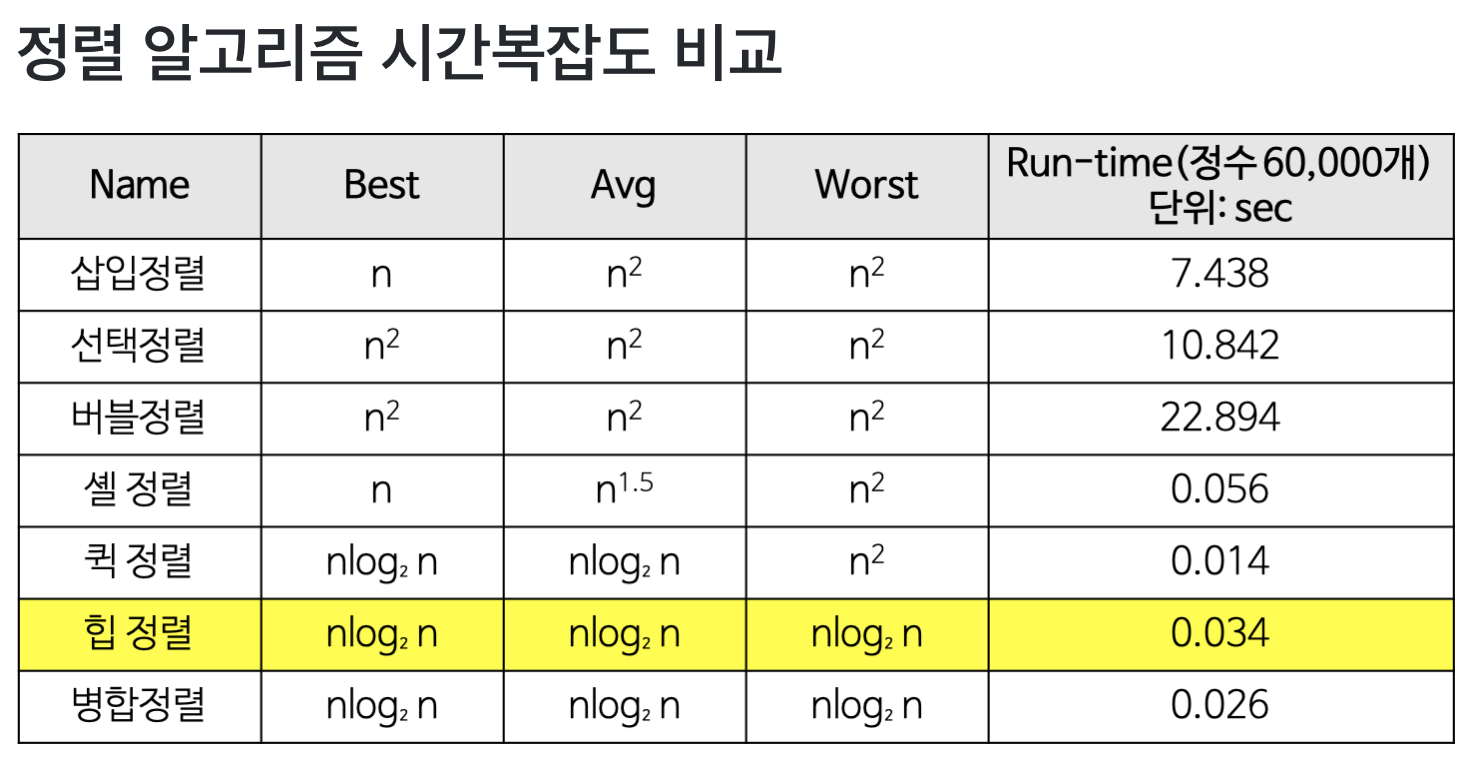
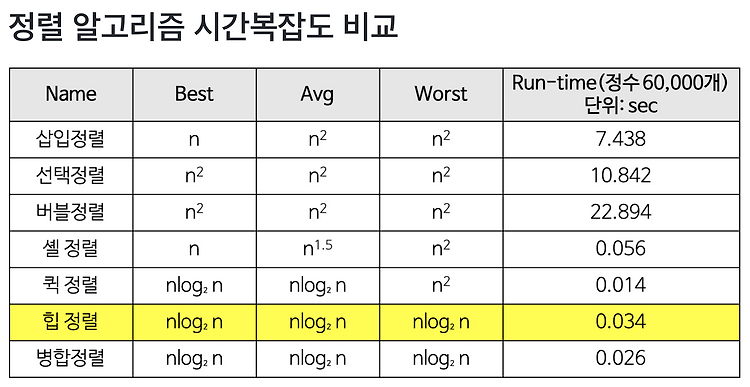
저희는 이제까지 Insertion sort(삽입 정렬), quck sort(퀵 정렬), merge sort(합병 정렬), heap sort(힙 정렬)에 대해 알아보았습니다. 이제까지 본 것 처럼 어느 한 상황에서 모두 다 뛰어 난 정렬 알고리즘은 존재하지 않습니다.
- 단순(구현 간단)하지만 비효율적인 방법
- 삽입정렬, 선택 정렬, 버블 정렬
- 복잡하지만 효율적인 방법
- 퀵 정렬, 힙 정렬, 합병 정렬, 기수 정렬
'School > DataStructure' 카테고리의 다른 글
| Data Structure - Sorting ( Bubble, Selection Sort ) (0) | 2022.05.29 |
|---|---|
| Data Structure - Sorting ( Several Keys && Radix Sort && External Sort ) (0) | 2022.05.28 |
| Data Structure - Sorting ( Merge Sort ) (0) | 2022.05.28 |
| Data Structure - Sorting ( Quick Sort ) (0) | 2022.05.28 |
| Data Structure - Sorting ( Insertion Sort ) (0) | 2022.05.28 |